This story comes from our special January 2021 issue, “The Beginning and the End of the Universe.” Click here to purchase the full issue.
In ancient times, scholars such as Aristotle thought that heavy objects would fall faster than lightweight objects under the influence of gravity. About four and a half centuries ago, Galileo Galilei decided to test this assumption experimentally. He dropped objects of different masses from the Tower of Pisa and found that gravity actually causes them all to fall the same way. More than 300 years later, Albert Einstein was struck by Galileo’s finding. He realized that if all objects follow the same trajectory under gravity, then gravity might not be a force but rather a property of space-time — the fabric of the universe, which all objects experience in the same way.
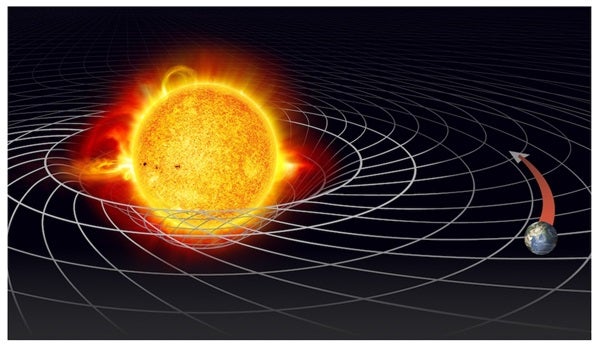
In one of the most important advances in modern physics, Einstein recognized that when space-time is curved, objects do not follow straight lines. He reckoned that Earth, for example, orbits the Sun in a circle because the Sun curves space-time in its vicinity. This is similar to the path of a ball on the surface of a trampoline whose center is weighed down by a person.
In November 1915, Einstein published the mathematical equations that established the foundation for his general theory of relativity. These equations describe the link between matter and the space-time in which it resides, showing that mass deforms space-time and influences the path of matter. In the words of physicist John Wheeler: “Space-time tells matter how to move and matter tells space-time how to curve.”
Schwarzschild’s solution
A few months later, while serving on the German front during World War I, Karl Schwarzschild became the first to derive a solution to Einstein’s equations. His solution describes the curved space-time around a point of mass, labeled by Wheeler half a century later as a “black hole.” Schwarzschild’s solution showed that the curvature of space-time diverges to infinity at the centermost point. This point is called the singularity because it is the singular point where Einstein’s theory breaks down.
The breakdown occurs because Einstein’s theory is missing a key component: quantum mechanics. Despite many attempts to unify general relativity with quantum mechanics (such as versions of string theory or loop quantum gravity), we do not have an experimentally verified version of the theory as of yet.
Gladly, the rest of space-time is protected from the uncertain description of the singularity. Schwarzschild’s solution provides a spherical event horizon that surrounds the singularity at the so-called Schwarzschild radius. The extent of this radius scales with the mass of the object within. No information can escape from inside this event horizon, which is why we cannot see down to the singularity of a black hole.
The fabric of the cosmos
But Einstein’s equations don’t solely apply to the space-time around a black hole. They also describe the evolution of the universe at large.
We know several facts from observing the universe over the past century. First, the universe is expanding. Second, on very large scales, the expanding universe is nearly homogeneous (meaning it has the same density of matter and radiation) and isotropic (meaning it has the same expansion rate in all directions).
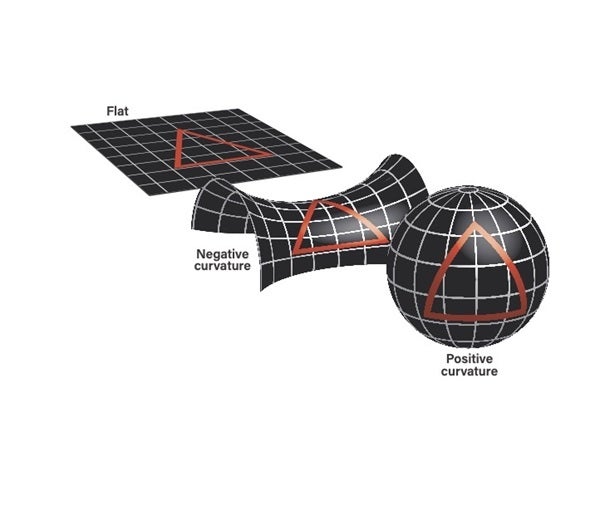
In the spirit of Galileo, can we measure the actual cosmic geometry experimentally? The simplest experimental approach is to draw a large triangle through the universe and measure the sum of its angles. For a negative or positive curvature, the sum would be smaller or larger than 180°, respectively, whereas for a flat geometry it would be exactly 180°.
The cosmos has been kind enough to embed the base of this triangle in the cosmic microwave background (CMB). Early on, the universe was hot and dense. The cosmic soup of particles cooled to a temperature below 4,000 Kelvin (about 6,700 degrees Fahrenheit or 3,700 degrees Celsius) 380,000 years after the Big Bang, at which point electrons and protons “recombined” to make hydrogen atoms and the universe became transparent to the CMB, allowing its light to travel unhindered. Therefore, observations of the CMB allow us to witness the universe at the moment of recombination.
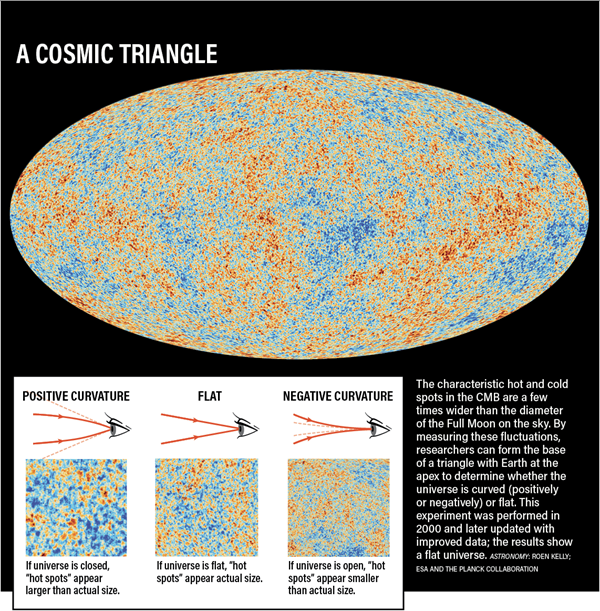
The CMB’s brightness is not perfectly uniform across the sky — it varies by roughly one part in 100,000 on a wide range of angular scales. But there is one special scale at the epoch of recombination which cosmologists can calculate: the distance that sound (acoustic) waves traversed over the course of these first 380,000 years of the universe. This acoustic scale can serve as the known base of our triangle. It signifies the spatial separation of parcels of the cosmic gas that could have been in acoustic contact with each other. By measuring this special correlation scale for CMB brightness fluctuations on the sky, we can draw an isosceles triangle with Earth at the apex. Knowing the height and base length of the triangle, as well as measuring the angle spanned by the acoustic scale on the sky, would tell us whether the sum of the angles in this triangle equals or deviates from 180° — and hence the curvature of the universe.
Our flat universe
Researchers performed this experiment in 2000 and later refined the measurement to a high level of precision with the latest data from the Planck satellite. The result revealed that the geometry of the universe is the simplest one we can imagine: flat!
Why is the universe so simple? Obviously, nature is under no obligation to represent the simplest solution to Einstein’s equations.
The theory of cosmic inflation provides one possible explanation. If the universe went through an early period during which it inflated exponentially, then all traces of its initial curvature would be flattened out. Inflation serves as the cosmic iron, erasing all pre-existing wrinkles from space-time. Quantum fluctuations of the vacuum during inflation might have led to the slight brightness fluctuations of the CMB that later seeded the formation of galaxies like the Milky Way. If our cosmic roots were formed then, we owe our existence to the quantum realm.
Interestingly, our expanding universe is now entering a new phase of exponential expansion, due to dark energy. Here again, we have no idea how long this inflationary phase will last. If it continues for more than 10 times the current age of the universe, our galaxy will be left alone, surrounded by darkness with no other source of light in sight. It would be the most dramatic incarnation of social distancing from extragalactic civilizations that we can imagine following the era of COVID-19.
Avi Loeb chairs the Board on Physics and Astronomy of the National Academies as serves as the founding director of Harvard’s Black Hole Initiative, and director of the Institute for Theory and Computation at the Harvard-Smithsonian Center for Astrophysics. His new book, Extraterrestrial (Houghton Mifflin Harcourt), is available from MyScienceShop.