Planets orbit their parent stars while separated by enormous distances – in our solar system, planets are like grains of sand in a region the size of a football field. The time that planets take to orbit their suns have no specific relationship to each other.
But sometimes, their orbits display striking patterns. For example, astronomers studying six planets orbiting a star 100 light years away have just found that they orbit their star with an almost rhythmic beat, in perfect synchrony. Each pair of planets completes their orbits in times that are the ratios of whole numbers, allowing the planets to align and exert a gravitational push and pull on the other during their orbit.
This type of gravitational alignment is called orbital resonance, and it’s like a harmony between distant planets.
I’m an astronomer who studies and writes about cosmology. Researchers have discovered over 5,600 exoplanets in the past 30 years, and their extraordinary diversity continues to surprise astronomers.
Harmony of the spheres
Greek mathematician Pythagoras discovered the principles of musical harmony 2,500 years ago by analyzing the sounds of blacksmiths’ hammers and plucked strings.
He believed mathematics was at the heart of the natural world and proposed that the Sun, Moon and planets each emit unique hums based on their orbital properties. He thought this “music of the spheres” would be imperceptible to the human ear.
Four hundred years ago, Johannes Kepler picked up this idea. He proposed that musical intervals and harmonies described the motions of the six known planets at the time.
To Kepler, the solar system had two basses, Jupiter and Saturn; a tenor, Mars; two altos, Venus and Earth; and a soprano, Mercury. These roles reflected how long it took each planet to orbit the Sun, lower speeds for the outer planets and higher speeds for the inner planets.
He called the book he wrote on these mathematical relationships “The Harmony of the World.” While these ideas have some similarities to the concept of orbital resonance, planets don’t actually make sounds, since sound can’t travel through the vacuum of space.
Orbital resonance
Resonance happens when planets or moons have orbital periods that are ratios of whole numbers. The orbital period is the time taken for a planet to make one complete circuit of the star. So, for example, two planets orbiting a star would be in a 2:1 resonance when one planet takes twice as long as the other to orbit the star. Resonance is seen in only 5% of planetary systems.
In the solar system, Neptune and Pluto are in a 3:2 resonance. There’s also a triple resonance, 4:2:1, among Jupiter’s three moons: Ganymede, Europa and Io. In the time it takes Ganymede to orbit Jupiter, Europa orbits twice and Io orbits four times. Resonances occur naturally, when planets happen to have orbital periods that are the ratio of whole numbers.
Musical intervals describe the relationship between two musical notes. In the musical analogy, important musical intervals based on ratios of frequencies are the fourth, 4:3, the fifth, 3:2, and the octave, 2:1. Anyone who plays the guitar or the piano might recognize these intervals.
Orbital resonances can change how gravity influences two bodies, causing them to speed up, slow down, stabilize on their orbital path and sometimes have their orbits disrupted.
Think of pushing a child on a swing. A planet and a swing both have a natural frequency. Give the child a push that matches the swing motion and they’ll get a boost. They’ll also get a boost if you push them every other time they’re in that position, or every third time. But push them at random times, sometimes with the motion of the swing and sometimes against, and they get no boost.
For planets, the boost can keep them continuing on their orbital paths, but it’s much more likely to disrupt their orbits.
Exoplanet resonance
Exoplanets, or planets outside the solar system, show striking examples of resonance, not just between two objects but also between resonant “chains” involving three or more objects.
The star Gliese 876 has three planets with orbit period ratios of 4:2:1, just like Jupiter’s three moons. Kepler 223 has four planets with ratios of 8:6:4:3.
The red dwarf Kepler 80 has five planets with ratios of 9:6:4:3:2, and TOI 178 has six planets, of which five are in a resonant chain with ratios of 18:9:6:4:3.
TRAPPIST-1 is the record holder. It has seven Earth-like planets, two of which might be habitable, with orbit ratios of 24:15:9:6:4:3:2.
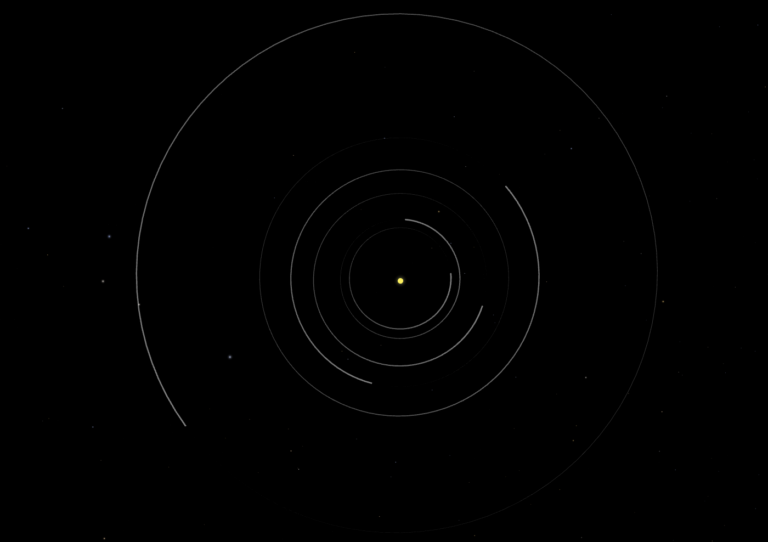
The newest example of a resonant chain is the HD 110067 system. It’s about 100 light years away and has six sub-Neptune planets, a common type of exoplanet, with orbit ratios of 54:36:24:16:12:9. The discovery is interesting because most resonance chains are unstable and disappear over time.
Despite these examples, resonant chains are rare, and only 1% of all planetary systems display them. Astronomers think that planets form in resonance, but small gravitational nudges from passing stars and wandering planets erase the resonance over time. With HD 110067, the resonant chain has survived for billions of years, offering a rare and pristine view of the system as it was when it formed.
Orbit sonification
Astronomers use a technique called sonification to translate complex visual data into sound. It gives people a different way to appreciate the beautiful images from the Hubble Space Telescope, and it has been applied to X-ray data and gravitational waves.
With exoplanets, sonification can convey the mathematical relationships of their orbits. Astronomers at the European Southern Observatory created what they call “music of the spheres” for the TOI 178 system by associating a sound on a pentatonic scale to each of the five planets.
A similar musical translation has been done for the TRAPPIST-1 system, with the orbital frequencies scaled up by a factor of 212 million to bring them into audible range.
Astronomers have also created a sonification for the HD 110067 system. People may not agree on whether these renditions sound like actual music, but it’s inspiring to see Pythagoras’ ideas realized after 2,500 years.
Chris Impey is a University Distinguished Professor of Astronomy at the University of Arizona.
This article was first published on The Conversation and is republished here under a Creative Commons license.